Derivation of magnetic field of wires

Sence the definition of the amp macroscopically depends on the magnetic field produced from a couple of pieces of wire, it is vital that the microscopic workings be well understood.
This can be done from the perspective of simple mechanics, involving classical laws.

e= coulomb of one electron
Density pa =free electrons in a cubic meter: n/m^3

I arbitrarily choose one amp and about 18 gauge wire because this is about the minimum wire size that can handle 1 amp without beginning to develop too much heat. I could have used triple-ot at 100amps too. In other words, drift speed is not likely to be much higher than this in copper wires - at any diameter.

Cross section wire Area: 17 AWG = 1.0 mm2 = 1E-7 m2
Amp: i = 1
i = dq/sec Amp: a = qe/sec = i * 6.2414504E18 e-charges/sec

N(Avogadro no): N = 6.022169E23 atoms per mole

Density Al: pg = 2.700g/cm3 = 2.70E3 kg/m3
atomic mass(Al): am = 26.981539 gm/mole = 26.981539E-3 Kg/mole
Density Al: pa = pg/am * N = (2.7E3/26.98E-3)N = 6.0266E28 Atoms/m3

Density Cu: pg = 8.933g/cm3 = 8.933E3 kg/m3
atomic mass(Cu): am = 64 gm/mole = 64E-3 Kg/mole
Density Cu: pa = pg/am * N = (8.9E3/64E-3)N = 8.4056306E28 Atoms/m3

Density Au: pg = 19.32g/cm3 = 19.32E3 kg/m3
atomic mass(Au): am = 196.96655 gm/mole = 196.96655E-3 Kg/mole
Density Au: pa = pg/am * N = (19.32E3/196.9E-3)N = 5.908E28 Atoms/m3

ALUMINUM

COPPER

GOLD

Although the drift-velocities are all different, the magnetic field from one amp of current is the same wether the wire is aluminum, copper, or gold. Drift velocity cancelled out in the equation leaving the magnetic field produced by a delta q/dt: a changing coulomb, dq/dt or current i. This is the nature of current in a "medium".

Derivation of Amperes-Law from Biot-Savart

RULERMAR.GIF, 1 kB

Biot-Savart B point charge
B equation with explicit velocity.

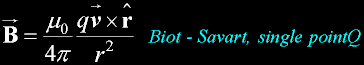
Magnetic field headed out of page at top, in blue, toward observer.

Biot-Savart B point charge

electron in motion

Each carrier (electron) in motion individually produces a magnetic field.

electrons in motion

Each electron in motion contributes to the magnetic field (label "B").

Biot-Savart B wire-current

Biot-Savart B wire-current
Using vectors...

coulomb pairs

Actually, I will use a simple non vector derivation...

Each coulomb of moving charge (shown in green) contributes to the magnetic field (shown in blue) .
Current in a "medium" is much better characterized by this formula.

wire

Each Current segment contributes to the magnetic field (label "B").
R=distance from wire in meters
D=Direct distance to each contributor of current
L=Length of wire from orthoganal of B

RULERYEL.GIF, 4 kB

Length of wire is infinite.

Due to symmetry...
Devide the integral into two parts...
1) neg-infinity to zero;
and
2) zero to pos-infinity

Substitute D

Substitute sin0

Form is ready to integrate

Generic indefinite integral equation from my book tables.
With L the variable and R a constant.

Definite integral evaluated.
Move out the constant R
to the coefficient

No meer constant (R) can compete with infinity; infinity always dominates.
And the limit therefor tends to L/L, or one.

A special case of amperes law
Of course, amperes law does not care about what caused the magnetic field. All that is needed is a "current" - What ever that means! It means that the current must be in a wire, and the wire must be the referance media.

Magnetic field B of an infinitley long wire...
RULERMAR.GIF, 1 kB

A charge traveling horizontally
Colored in false-color representing a dimension of B intensity.
Picture a knife slicing down through a doughnut that is standing up, edge-on toward the observer.

(As far as color goes, it looks like I totally stop graphing in one color (red) before picking up the next (green). Oh well... it still shows the geometry (dramatically.
I think it is a type of toroid (in three dimensions).)

A point-charge (in a small section of wire);
changing horizontally at 1 coulomb per sec.
The wire has a radius of near zero meters.

EqnBCUR2.GIF, 1 kB

Graduations are in tenths of a meter from the point-charge(s) .1m
Red circle is a magnetic field strength of2*u Tesla = 2.513E-6 T.

If electric charges are moving to the right, and/or holes moving to the left, then
magnetic field lines circle and approach toward observer in the bottom section (y less than 0) and reseed in the top section (away from observer).

EqnSUMe.gif, 1 kB

I have shown there is an equivalence between q/sec in a wire, and that of drift speed of isolated particles.

I have also shown that speeds are far below relativistic speeds. No need to invoke Einstein. Everything works perfectly - as is. It is absolutely Classical and simple.

But I am sure Einstein went down this same road...
I have shown two force equations. One the force from moving charges relative to a "stationary" magnetic field. The other the force from a changing magnetic field relative to stationary charges. Both are absolutely profoundly empirical. Both describe the same thing from two relatively different positions. Yet they are different equations. They MUST be equal! From these two Classical - and almost Mechanical - equations we can derive the Lorentz contraction observation. And, I think, venture into the world of Einstein.

The dual monopoles:
I have shown that macroscopically a moving electrical charge in a wire only exists as a dual electrical monopoles. Each group of charges have a relative speed to the wire media. And they are statistically equal in number.

In fact, I would continue with the notion that a photon itself is constituted by two monopoles. I would extend the concept to guess at the internal workings of a photon: Two electrostatic monopoles of opposite charge oscillate back and forth in a polarization plane. They seem to pass through or "add" to each other at the line of forward motion.

I have also demonstrated that forces of moving isolated single charges experience a force to a non moving magnetic field. I have demonstrated that this is the same force as static non moving current carrying wire experienced by a changing, or moving magnetic field. The latter forms the definition of current in amps, and has been my verification.